Understand Domain, Range, and Harmonic Progression in Detail

Functions is a building block for calculus and other high-weightage topics and hence a very important chapter in the preparation of competitive entrance exams. Students must know about the different types of functions and their use along with how to find the Domain and Range of a Function.

SSC Delhi Police Constable Online Form 2020 - Post 551

Sequence and series are two of the most common subjects in banking exams. These pattern-based progressions can be Arithmetic, Geometric and Harmonic Progressions. Most students are well acquainted with arithmetic and geometric progression since elementary school. It is Harmonic progression series that they get familiar with later in life or maybe while preparing for a competitive exam. Some important concepts to focus on in Harmonic Progression includes Harmonic Progression Formula, Sum of Harmonic Progression, and Harmonic Mean.

On that note, let’s discuss the basic concepts of Domain, Range, and Harmonic Progression useful for students appearing for competitive exams.

Domain and Range

Based on the likelihood of the provided function being defined in the real set, the domain and range of a function can be specified. Let’s take a closer look at Domain and Range, which are described in-depth here.

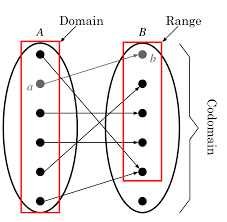
Domain

The domain of a function is the set of all potential values that qualify as inputs to a function. It can alternatively be described as the full set of values available for independent variables.

Assume we establish a relation F from set A to B that correlates countries with the year in which they first won the World Cup. As a result, each element in set A will be precisely connected with only one element in set B.

Where SetA={India, Pakistan, Australia, Sri Lanka} and SetB= {1975,1979, 1983, 1987, 1992, 1996, 1999, 2003, 2007, 2011, 2015}

For example, the domain of the function F is set to A, i.e., {India, Pakistan, Australia, and Sri Lanka}.

Finding the Domain of a function

Examine the values of the independent variables that can be used to establish the domain, i.e. no zero at the bottom of the fraction and no negative sign inside the square root.

Subject to specific constraints, the domain of a function is often defined as the set of all real numbers (R).

They are as follows:

When the supplied function has the form f(x) = 2x + 5 or f(x) = x2 – 2, the domain is “the set of all real numbers.”

The domain is the set of all real numbers except one when the supplied function is of the type f(x) = 1/(x – 1).

Range

The range of a function is the set of all the outputs of a function, or, after replacing the domain, the whole set of all values imaginable as dependent variable outcomes.

For example. the range of the function F is {1983, 1987, 1992, 1996}.

Finding the Range of a Function

Consider the following function: y = f (x).

The range of the function is the spread of all the y values from least to maximum.

Substitute all the values of x in the provided expression of y to see whether it is positive, negative, or equal to other values.

Determine the minimum and maximum y values and draw the graph.

Harmonic Progression

A Harmonic Progression (HP) is a sequence of real numbers formed by calculating the reciprocals of the arithmetic progression that does not contain 0. The sequence a, b, c, d,…, for example, is regarded as an arithmetic progression; the harmonic progression can be represented as 1/a, 1/b, 1/c, 1/d,…

Harmonic Mean

The harmonic mean is calculated using the reciprocal of the arithmetic mean of the reciprocals. The formula to find the harmonic mean is as follows:

Harmonic Mean = n /[(1/a) + (1/b)+ (1/c)+(1/d)+….]

The Nth Term and Harmonic Progression Sum Formula

The formula for determining the nth term of the harmonic progression series is as follows:

The nth term in a Harmonic Progression = 1/ [a+(n-1)d]

The formula for calculating the sum of n terms in a harmonic progression is as follows:

Sum of n terms,

S_n= 1/d ln { 2a + (2a-1)d/ 2a-d}

Read More:

Sarkari Results